Question

Two horizontal forces act on a 5.0-kg mass. One force has a magnitude of 8.0 N and is directed due north. The second force toward the east has a magnitude of 6.0 N. What is the acceleration of the mass?

Answer 1

The acceleration of the mass is 2 m/s² and its direction is 53.1° north of east.

Step-by-step explanation:

Hi there!

Let´s consider the horizontal plane with coordinates (x,y) (see attached figure).

The resulting force acting on the mass is the sum of the north and east vectors.

The east force vector will be:

F east = (6.0 N, 0)

while the north force vector will be:

F north = (0, 8.0 N)

Then, the resulting vector acting on the mass will be:

F = F east + F north = (6.0 N, 0) + (0, 8.0 N) = (6.0 N + 0, 0 + 8.0 N) = (6.0, 8.0) N

The acceleration of the mass will be a vector with an x and y-component. Each component can be obtained from the equation of force:

F = m · a

Where:

m = mass of the object.

a = acceleration.

Then:

a = F/m

The x-component (east component) of the acceleration will be:

ax = Fx/m

ax = 6.0 N / 5.0 kg = 1.2 m/s²

The y-component of the acceleration will be:

ay = Fy/m

ay = 8.0 N /5.0 kg

ay = 1.6 m/s²

Then, the acceleration of the mass will be the vector (1.2, 1.6) m/s².

The magnitude of the acceleration will be:

`|a| = \sqrt{(1.2 m/s^(2))^(2) + (1.6 m/s^(2))^(2)} = 2 m/s^(2)`

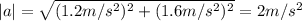
Then the acceleration will be 2 m/s²

We can also find the direction of the acceleration vector using trigonometry:

cos θ = adjacent / hypotenuse

cos θ = 1.2/2

θ = 53.1°

Then, the mass is accelerated in a direction 53.1° north of east.