Question

A newspaper conducted a statewide survey concerning the 1998 race for state senator. The newspaper took a SRS of n=1400 registered voters and found that 720 would vote for the Republican candidate. Let p represent the proportion of registered voters in the state who would vote for the Republican candidate.

We test

H0:p=.50
Ha:p>.50

(a) What is the z -statistic for this test?

(b) What is the P-value of the test?

Answer 1

Answer: a) 1.07, b) 0.1423

Explanation:

Since we have given that

n = 1400

Number of voters vote for Republican candidates = 720

So,
`\hat{p}=(720)/(1400)=0.514286`

And hypothesis are :

`H_0:p=0.50\\\\H_a:p>0.50`

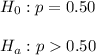
(a) What is the z -statistic for this test?

`z=\frac{\hat{p}-p}{\sqrt{(p(1-p))/(n)}}\\\\z=\frac{0.514286-0.50}{\sqrt{(0.50* 0.50)/(1400)}}\\\\z=1.06609\approx 1.07`

(b) What is the P-value of the test?

p-value = P(Z>Z(statistics))

`P(z>1.07)\\\\=0.1423`

Hence, a) 1.07, b) 0.1423