Question

Air is compressed by an adiabatic compressor from 95 kPa and 27°C to 600 kPa and 277°C. Assuming variable specific heats and neglecting the changes in kinetic and potential energies, determine (a) the isentropic efficiency of the compressor, and (b) the exit temperature of the air if the process were reversible.

Answer 1

isentropic efficiency = 0.818

Step-by-step explanation:

given data

pressure P1 = 95 kPa

temperature = 27°C

pressure P2 = 600 kPa

temperature = 277°C

to find out

isentropic efficiency of the compressor and exit temperature of the air

solution

we know from ideal gas of properties of air is

Pr1 at 27°C = 1.3860

and h1 at 300 K = 300.19 kJ/kg

and h2 at 550 K = 555.74 kJ/kg

and

we know equation for isentropic process that is

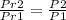`(Pr2)/(Pr1) =(P2)/(P1)` .........................1

put here value we get

`(Pr2)/(1.3860) =(600)/(95)`

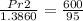
solve we get Pr2

Pr2 = 8.75

by ideal gas of properties of air will be at Pr2

h2s = 508.66

T2s = 505.5 K

'so

isentropic efficiency will be here as

isentropic efficiency =
`(h2s-h1)/(h2-h1)`

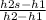
isentropic efficiency =
`(508.66-300.19)/(555.74-300.19)`

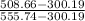
isentropic efficiency = 0.818