Answer:
h≅ 58 m
Step-by-step explanation:
GIVEN:
mass of rocket M= 62,000 kg
fuel consumption rate = 150 kg/s
velocity of exhaust gases v= 6000 m/s
Now thrust = rate of fuel consumption×velocity of exhaust gases
=6000 × 150 = 900000 N
now to need calculate time t = amount of fuel consumed÷ rate
= 744/150= 4.96 sec
applying newton's law
M×a= thrust - Mg
62000 a=900000- 62000×9.8
acceleration a= 4.71 m/s^2
its height after 744 kg of its total fuel load has been consumed
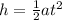
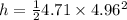
h= 58.012 m
h≅ 58 m