Question

Given: x + 2 < -5.

Choose the solution set.
A. x R, x < -7
B.x
C.x
D.x

Answer 1

Choice A. {
`x|x\,\epsilon\,R, x\,<\,-7` }

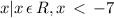Explanation:

Start by working on isolating the variable "x" in the inequality
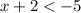`x+2<-5`. We can do such by subtracting "2" from both sides:

`x+2<-5\\x+2-2<-5-2\\x<-7`

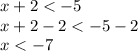
Therefore, we need to consider all those real values of the variable "x" which are strictly smaller than -7 (reside to the left of -7 on the number line).

Such set of x-values can be described in set notation (the notation suggested in your answer choices) specifying:

a) with the group symbols "{" and "}" the beginning and the end respectively of the set to be described,

b) with the expression "x |" we state: all those x-values such that,

c) with the symbols
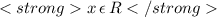`<strong>x\,\epsilon\,R</strong>` we state: x belongs to the set of Real (R) numbers,

d) and finally the condition on the values allowed for the variable x: x < -7 (x values must be strictly smaller than "-7"

Answer 2

A. {x/x R, x< -7}

Explanation:

x+ 2 < -5 becames x < -7 by subtracting -2 at both sides of the inequation. Because inequations mantain the same direction when the operation of sum or subtraction is applied, the results yiels x< -7-