Question

Assume that the heights of boys in a high school basketball tournament are normally distributed, with mean 70 inches and standard deviation 2.5 inches. What is the expected number of boys in a group of 40 who are taller than 70 inches?

Answer 1

Answer: 20

Explanation:

We assume that the heights of boys in a high school basketball tournament are normally distributed.

Given : Mean height of boys :
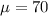`\mu=70` inches.

Standard deviation:
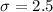`\sigma=2.5` inches.

Let x denotes the heights of boys in a high school basketball tournament .

Then the probability that a boy is taller than 70 inches will be :-

`P(x> 70)=1-P(x\leq70)\\\\=1-P((x-\mu)/(\sigma)\leq(70-70)/(2.5))\\\\=1-P(z\leq0)=1-0.5=0.5\ \ \text{[by using z-value table]}`

Now, the expected number of boys in a group of 40 who are taller than 70 inches will be :-

`40*0.5=20`

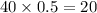
Hence, the expected number of boys in a group of 40 who are taller than 70 inches=20