Question

Sterile fruit flies are used in an experiment where the proportion that survives at least t days is given by e^−0.15t. If the experiment begins with 400 fruit flies, and flies are added at the rate of 6 per hour, how many flies are present 15 days after the start of the experiment? (Round your answer to the nearest integer.)

Answer 1

901 flies

Explanation:

Let the total population of flies is P,

While
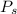`P_s` and
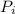`P_i` represent the number of flies that survived and that are initially respectively.

Here,

`(P_s)/(P_i)=e^(-0.15t)`

`\implies P_s = P_i e^(-0.15t)`

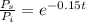
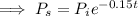
Differentiating w. r. t. t ( time ),

`(dP_s)/(dt)=-0.15 P_i e^(-0.15t)=-0.15 P_s-----(1)`

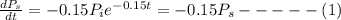
Now, if 6 flies are added per hour,

Then the number of flies added per day = 24 × 6 = 144 ( ∵ 1 day = 24 hours),

So,

`(dP)/(dt)=144 + (dP_s)/(dt)`

`(dP)/(dt)=144 -0.15 P_s`

`\implies (dP)/(dt)=144 -0.15 P`

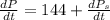
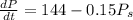
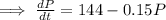
When P =
`P_s` =
`P_i`

`\implies (dP)/(144 -0.15 P)=dt`

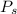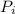
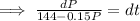
∵ if t = 0, P = 400,

`\int_(400)^(P)(dP)/(144 -0.15 P)=\int_(t=0)^(15)dt`

`[(\ln(144-0.15P))/(-0.15)}]_(400)^(P) = [t]_(0)^(15)`

`(\ln (144-0.15P)- \ln(144-60))/(-0.15)}=15`

`\ln (144-0.15P) - \ln(84) = -2.25`

`-\ln (144-0.15P) + \ln(84) = 2.25`

`\ln ((84)/(144-0.15P))= 2.25`

`(84)/(144-0.15P) = e^(2.25)`

`(84)/(e^(2.25)) =144-0.15P`

`8.853 = 144 - 0.15P`

`0.15P = 144 - 8.853`

`\implies P = (135.147)/(0.15)=(135.147)/(0.15)\approx 901`

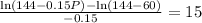
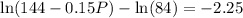
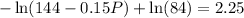
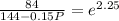
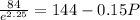
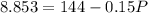
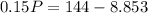
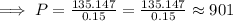
i.e. 901 flies would present.