Question

The weights of newborn babies are distributed​ normally, with a mean of approximately 105 oz and a standard deviation of 10 oz. If a newborn baby is selected at​ random, what is the probability that the baby weighs more than 75 ​oz

Answer 1

Answer: 0.9987

Explanation:

Given : The weights of newborn babies are distributed​ normally, with a mean of approximately 105 oz and a standard deviation of 10 oz.

i.e.
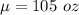`\mu=105\ oz` and
`\sigma= 10\ oz`

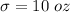
Let x represents the weights of newborn babies.

If a newborn baby is selected at​ random, then the probability that the baby weighs more than 75 ​oz will be :-

`P(x>75)=P((x-\mu)/(\sigma)>(75-105)/(10))\\\\ =P(z>-3 )=P(z<3)\ \ \ [\because\ P(Z>-z)=P(Z<z)]`

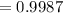`=0.9987` [using z-value table]

Hence, the required probability = 0.9987