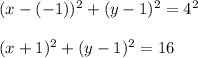Question

Which equation represents a circle with the same radius as the circle shown but with a center at (-1, 1)? (x – 1)2 + (y + 1)2 = 16 (x – 1)2 + (y + 1)2 = 4 (x + 1)2 + (y –1)2 = 4 (x + 1)2 + (y – 1)2 = 16

Answer 1

`\displaystyle (x + 1)^2 + (y - 1)^2 = 16`

Step-by-step explanation:

According to the Center-Radius Formula,
`\displaystyle [X - H]^2 + [Y - K]^2 = R^2,`[H, K] represents the centre of the circle, where the negative symbols give the OPPOSITE terms of what they really are, and the radius is ALWAYS squared. So, with the centre of
`\displaystyle [-1, 1]`plus your radius of 4, you have this:

`(x + 1)^2 + (y - 1)^2 = 16`

I am joyous to assist you anytime.

Answer 2

Last option:
`(x+1)^2+(y-1)^2=16`

Explanation:

The missing figure is attached.

The center-radius form of the circle equation is:

`(x - h)^2 + (y-k)^2 = r^2`

Where the center of the circle is at the poitn
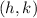`(h,k)` and "r" is the radius.

You can identify from the figure attached that the radius of the circle shown is 4 units.

Since the other circle has the same radius and its center is at the point
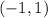`(-1, 1)`; you can identify that:

`h=-1\\k=1\\r=4`

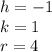
Therefore, substituting values into
`(x - h)^2 + (y-k)^2 = r^2`, you get that the equation of that circle is:

`(x - (-1))^2 + (y-1)^2 = 4^2\\\\(x+1)^2+(y-1)^2=16`