Question

Points A and B are on opposite sides of a lake. A point C is 81.3 meters from A. The measure of angle BAC is 78.33°, and the measure of angle ACB is determined to be 34.167°. Find the distance between points A and B (to the nearest meter).

Answer 1

82 yards

Explanation:

I got it correct on founders edtell

Answer 2

The distance between the points A and B is 49 meters.

Explanation:

Here we have to use "The law of sines" and find distance between the points A and B.

Law of sines =
`(a)/(sin A) = (b)/(sin B) = (c)/(sin C)`

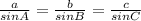
Where a, b, c are the sides of a triangle and A, B, and C are the angles of the triangle.

First let's draw the figure of the given situation.

From the given information, we can form a triangle ABC.

We are given two angles. We can find the third angle ABC

The sum of the three angles add upto 180 degrees.

78.33 + 34.167 + ∠ABC = 180

112.497 + ∠ABC = 180

∠ABC = 180 - 112.497

∠ABC = 67.503°

So, ∠A = 78.33° , ∠B = 67.503° and ∠C = 34.167°

We know that side AC = b = 81.3 m

Now we can use the law of sine and find the length AB.

In the triangle, AB is called the side c which is opposite to the angle C.

AC is called the side b which is opposite to the angle B.

\frac{b}{sin B} = \frac{c}{sin C}[/tex]

`(81.3)/(sin 67.503) = (AB)/(sin 34.167)`

Now we can find the value of sin 67.503 and sin 34.167 using the calculator, we get

`(81.3)/(0.924) = (AB)/(0.562)`

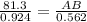
Cross multiplying, we get

0.924 × AB = 81.3 × 0.562

AB = 45.6906 ÷ 0.924

AB = 49.45 meters

When we round off to nearest meters, we get

AB = 49 meters.

Therefore, the distance between the points A and B is 49 meters.