Question

I need help please with my questions.

Answer 1

1. (4a+3)(4a-3)

2. (2m + 5)(2m-5)

3. (4b-5)²

4. (2x-1)²

5. (3x+1)(3x-1)

6. (n+5)(n-5)

7. (n²-10)²

8. (a²+3)(a²-3)

9. (k²+6)(k²-6)

10. (n²+7)(n²-7)

Explanation:

For special products like these, just remember the

Difference of Squares formula:

`a^2 -b^2 = (a+b)(a-b)`

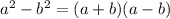
and

Square of difference formula:

`a^2-2ab+b^2=(a-b)²`

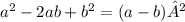
1. 16a²-9 follows the difference of squares form:

a² - b² = (a+b)(a-b)

So all we need to do is get the square root of a and b and follow the form:

(√16a²) - (√9) = (4a +3) (4a - 3)

Let's check through FOIL:

(4a +3) (4a - 3)

16a² - 12a + 12a - 9

16a² - 9

2. 4m² - 25 also follows the same form of difference of squares:

(√4m²) - (√25) = (2m +5) (2m - 5)

Let's check!

(2m +5) (2m - 5)

4m² + 10m - 10m -25

4m² - 25

3. 16b² - 40b + 25 follows the form of square of difference:

a² - 2ab + b² = (a - b)²

√16b² = 4b

√25 = 5

(4b - 5)²

Let's check!

(4b-5)(4b - 5)

16b² - 20b - 20b + 25

16b² - 40b + 25

HEre's another way to look at it:

First multiply the coefficients of the first and last term:

16 x 25 = 400

Next think of two numbers that when you multiply them, you will come up with 400 and sum up to -40. The two numbers would be -20 and -20 which will be the middle term

So it would fill in this way:

16b² - 20b - 20b + 25

Factor the equation:

(16b² - 20b) (-20b + 25)

4b(4b-5) - 5(4b-5)

(4b-5)(4b-5) = (4b-5)²

4. 4x² - 4x + 1 follows that same form:

√4x² = 2x

√1 = 1

(2x - 1)²

Let's check!

(2x - 1)(2x - 1)

4x² - 2x - 2x + 1

4x²- 4x + 1

5. 9x² - 1 follows the form of difference of squares:

√9x² - √1 = (3x + 1)(3x - 1)

Let's check!

(3x + 1)(3x - 1)

9x² + 3x - 3x - 1

9x² - 1

6. n² - 25 follows the form of difference of squares:

√n² - √25 = (n + 5)(n - 5)

Let's check!

(n + 5)(n - 5)

n² + 5n - 5n - 25

n² - 25

7. n⁴ - 100

√n⁴ - √100 = (n² + 10)(n² - 100)

Let's check!

(n² + 10)(n² - 100)

n⁴ + 10n² - 10n² -100

n⁴ - 100

8. a⁴ - 9

√a⁴ - √9 = (a² + 3)(a² - 3)

Let's check!

(a² + 3)(a² - 3)

a⁴ + 3a² - 3a² - 9

a⁴ - 9

9. k⁴ - 36

√k⁴ - √36 = (k² + 6) (k² - 6)

Let's check!

(k² + 6) (k² - 6)

k⁴ + 6k² - 6k² - 36

k⁴ - 36

10. n⁴ - 49

√n⁴ - √49 = (n² + 7) (n² - 7)

Let's check!

(n² + 7) (n² - 7)

n⁴ +7n² - 7n²- 49

n⁴ - 49