Question

1. Autoclaves that are used to sterilize surgical tools require a temperature of 120 oC to kill bacteria. If water is used, at what pressure must the autoclave operate? Hvap = 40.656 kJ/mol.

Answer 1

An autoclave must operate at a pressure close to the standard range of 15 to 20 psi to sterilize surgical tools at 120°C with water, due to the relation of pressure and boiling point as described by the Clausius-Clapeyron equation.

Step-by-step explanation:

The question asks at what pressure must an autoclave operate if water is used to sterilize surgical tools at a temperature of 120°C, given a heat of vaporization (Hvap) of 40.656 kJ/mol. To answer this, we refer to the Clausius-Clapeyron equation, which relates the pressure and temperature for a phase change, such as boiling. As standard autoclaves function at around 121°C at a pressure of 15 to 20 psi, achieving a temperature of 120°C would require the autoclave to operate at a pressure close to this range. This is due to water boiling at higher temperatures when the pressure is increased, which allows the steam to effectively sterilize instruments, including the resilient endospores.

Answer 2

The autoclave must operate at 1.94 atm pressure.

Step-by-step explanation:

To calculate
`P_2` of the reaction, we use Clausius Clapeyron equation, which is:

`\ln((P_2)/(P_1))=(\Delta H_(vap))/(R)[(1)/(T_1)-(1)/(T_2)]`

where,

`P_1` = Pressure of autoclave at temperature
`T_1` = 1 atm

`P_2` = Pressure of autoclave at temperature
`T_2` = ?

`\Delta H_(vap)` = Enthalpy of vaporization = 40.656 kJ/mol = 40,656 J/mol

R = Gas constant = 8.314 J/mol K

`T_1` = initial temperature =
`373.12 K`

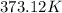
`T_2` = final temperature =
`120^oC=[120+273.15]K=393.15 K`

Putting values in above equation, we get:

`\ln((P_2)/(1 atm))=(40,656 J/mol)/(8.314J/mol.K)[(1)/(373.12 K)-(1)/(393.15 K)]\\\\ P_2=1.94 atm`

The autoclave must operate at 1.94 atm pressure.