Question

Solve for y. Enter your answer in the box. y = An equilateral triangle A B C. Side A B is the base. Sides A B, B C, and A C are equal. Sides A B, B C, and A C are labeled with single tick marks. Angle B is labeled as left parenthesis 3 x minus 3 right parenthesis degrees, and angle C is labeled as left parenthesis 8 y minus 4 right parenthesis degrees.

Answer 1

60 is not the answer. It's 8.

Explanation:

Answer 2

y = 8 And ∠A = ∠B = ∠C = 60°

Explanation:

Given ABC is an equilateral triangle having AB=BC=AC.

∠A = ∠B = ∠C

∠B = 3x-3 ∠C = 8y-4

∴ ∠A = 3x-3

We know that the sum of all the angles of a triangle is 180°.

`Solution\ 8y-4=3x-3=8y-3x=1 \ equation\ 1\\</p><p>3x-3+3x-3+8y-4=180`

`3x-3+4y-2=90\\3x+4y=95\ equation\ 2`

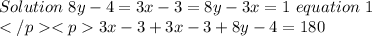
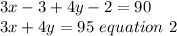
Now adding equation 1 and 2 we get ;

`12y=96\\y=8`

Now substituting the value of y we get measure of ∠C.

`\angle C = 8y-4 = 8 * 8 - 4 = 60\°`

∴∠A = ∠B = ∠C = 60°