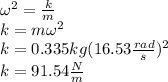Question

A 0.335 kg mass is attached to a spring and executes simple harmonic motion with a period of 0.38 s. The total energy of the system is 2.1 J. Find the force constant of the spring. Answer in units of N/m.

Answer 1

`k=91.54 (N)/(m)`

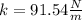
Step-by-step explanation:

The angular speed is defined as the angle traveled in one revolution over the time taken to travel it, that is, the period. Therefore, it is given by:

`\omega=(2\pi)/(T)\\\omega=(2\pi)/(0.38s)\\\omega=16.53(rad)/(s)`

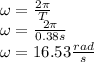
The angular frequency of the simple harmonic motion of the mass-spring system is defined as follows:

`\omega=\sqrt{(k)/(m)}`

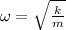
Here, k is the spring's constant and m is the mass of the body attached to the spring. Solving for k:

`\omega^2=(k)/(m)\\k=m\omega^2\\k=0.335kg(16.53(rad)/(s))^2\\k=91.54 (N)/(m)`