Answer:
0.0547,0.0078,0.25
Explanation:
Any random customer selected probability to recognize = 50% = 0.50 which remains the same for each customer. Also there are only two outcomes. Hence X the no of customers who recognize brand name is Binomial
X is Bin (7,0.50)
a)

b)
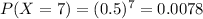
c)
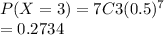
d) Here n changes from 7 to 4. But p probability for success remains the same.
Hence

This probability is not unusually high.
If 4 consumers are randomly selected is 3 an unusually high number of consumers that recognize the brand name is 0.25