Question

Given a || b , and c is not parallel to a or b,

which statements must be true?
Select each correct answer.
m<4=m<8
m<7=m<10
m<8 = m<29
m<2=m<7

Answer 1

m<4=m<8

m<2=m<7

Answer 2

Options A and D.

Explanation:

Given information: a || b , and c is not parallel to a or b.

If two lines are parallel and a transversal line intersect both lines, then

1. Corresponding angles are equal.

2. Alternate exterior angles are equal.

3. Alternate interior angles are equal.

Using the above definitions, we get

`\angle 4=\angle 8` (Corresponding angles)

`\angle 2=\angle 7` (Alternate exterior angles)

Since line c is not parallel to a or b.

`\angle 7=\angle 10`

`\angle 8=\angle 9`

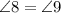
Hence, the correct options are A and D.