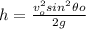Answer:
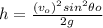
Step-by-step explanation:
The parabolic movement results from the composition of a uniform rectilinear motion (horizontal ) and a uniformly accelerated rectilinear motion of upward or downward motion (vertical ).
The equation of uniform rectilinear motion (horizontal ) for the x axis is :
x = xi + vx*t Equation (1)
Where:
x: horizontal position in meters (m)
xi: initial horizontal position in meters (m)
t : time (s)
vx: horizontal velocity in m/s
The equations of uniformly accelerated rectilinear motion of upward (vertical ) for the y axis are:
y= y₀+(v₀y)*t - (1/2)*g*t² Equation (2)
vfy= v₀y -gt Equation (3)
vfy²= v₀y²-2gH Equation (4)
Where:
y: vertical position in meters (m)
y₀ : initial vertical position in meters (m)
t : time in seconds (s)
v₀y: initial vertical velocity in m/s
vfy: final vertical velocity in m/s
g: acceleration due to gravity in m/s²
H= hight that reaches the projectile above its starting point (m)
Data
v₀ : total initial speed
θ₀ : angle of v₀ above the horizontal.
g acceleration due to gravity
Calculation of the componentes x-y of the v₀
v₀x = v₀*cos θ₀
v₀y = v₀*sin θ₀
Calculation of the maximum hight that reaches the projectile above its starting point
When the projectile reaches its maximum height (h), vy = 0:
in the Equation (4)
vfy²= v₀y²-2gh
0= v₀y²-2gh
2gh= v₀y²
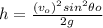
2gh= (v₀*sin θ₀)²