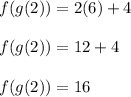Question

F(n)=2n+4 g(n) = 4n - 2 Find f (g(2))

Answer 1

Answer:
`f(g(2))=16`

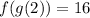
Explanation:

Given the function
`f(n)`:

`f(n)=2n+4`

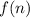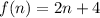
And the function
`g(n)`:

`g(n) = 4n - 2`

The first step we can apply is to find
`g(2)`. To do it, we need to substitute
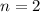`n=2` into the function and then we must evalute. Then, this is:

`g(2) = 4(2) - 2\\\\g(2) =8 - 2\\\\g(2)= 6`

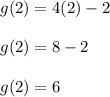
Finally, in order to find
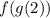`f (g(2))` we need to substitute
`g(2)` found above, into the function
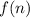`f(n)` and then we must evaluate.

So, we get:

`f(g(2))=2(6)+4\\\\f(g(2))=12+4\\\\f(g(2))=16`