Question

The greatest common factor of 16 and the positive integer n is 4, and the greatest common factor of n and 45 is 3. Which of the following could be the greatest common factor of n and 210?

Answer 1

6 if 7 doesnt divide n, 42 otherwise. The only possible options are those 2.

Explanation:

I will find the possible answers, since no options were given

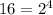`16 = 2^4` , thus, 16 only has 2 as a prime divisor. We conclude that n = 4*k, with k an odd number. On the other hand,
`45 = 3^2 * 5` , since the greatest factor between n and 45 is 3, then 5 is not a divisor of n, and n has the form 3*j, with j not a multiple of 3. Since 3 and 4 doesnt have common primes as factors, then j = 4*l, with l odd.

Thus n = 12 * j, with j a number that satisfies:

• 3 is not a divisor of j
• 2 is not a divisor of j
• 5 is not a divisor of j

Note that
`210 = 2*3*5*7` . 12 is not factor of 210, but 6 is. j doesnt have any prime divisor in common with 210, with the exception, maybe, of 7. So the greatest common factor of them is 1 or 7, depending on weather 7 divides j (or equivalently, n) or not. Therefore, the greatest common factor of n and 210 could be 6, if 7 does not divide n, or 6*7 = 42, if 7 divides n.

I hope that works for you!