Answer: x= -2
Explanation:
Given the equation
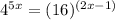
Now, we can also write the above equation as
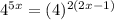
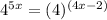
Now, taking log on both sides
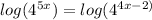
So, the above the equation can be written as
5x=4x-2
Simplifying the equation,
5x-4x=2
x= -2
Hence, the value of x = -2. Which satisfy the given equation in the question.