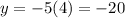Question

1) If y varies inversely as x, and y=3 as x = -2, find y for the x value of -1.

2) If y varies directly as x, and y=15 as x=-3, find y for the x value of 4

Answer 1

Part 1) y=6

Part 2) y=-20

Explanation:

Part 1) If y varies inversely as x, and y=3 as x = -2, find y for the x value of -1.

we know that

A relationship between two variables, x, and y, represent an inverse variation if it can be expressed in the form
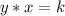`y*x=k` or
`y=k/x`

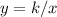
we have

For x=-2, y=3

Find the value of the constant k

`k=y*x`

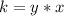
substitute the values of x and y

`k=(3)(-2)=-6`

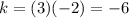
The equation is equal to

`y=-6/x`

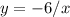
so

For x=-1

substitute in the equation and solve for y

`y=-6/(-1)=6`

Part 2) If y varies directly as x, and y=15 as x=-3, find y for the x value of 4

we know that

A relationship between two variables, x, and y, represent a proportional variation if it can be expressed in the form
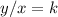`y/x=k` or
`y=kx`

we have

For x=-3, y=15

Find the value of the constant k

`k=y/x`

substitute the values of x and y

`k=15/-3=-5`

The equation is equal to

`y=-5x`

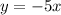
so

For x=4

substitute in the equation and solve for y

`y=-5(4)=-20`