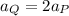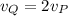Question

A horizontal disk rotates about a vertical axis through its center. Point P is midway between the center and the rim of the disk, and point Q is on the rim. If the disk turns with constant angular velocity, which of the following statements about it are true? (There may be more than one correct choice.)

P and Q have the same linear acceleration.
The angular velocity of Q is twice as great as the angular velocity of P.
The linear acceleration of P is twice as great as the linear acceleration of Q.
The linear acceleration of Q is twice as great as the linear acceleration of P.
is moving twice Q as fast as P.

Answer 1

The linear acceleration of Q is twice as great as the linear acceleration of P.

Step-by-step explanation:

As we know that disc is rotating about the vertical axis with constant angular speed

So here we can say that the acceleration of any point on the disc is given as

`a = \omega^2 r`

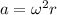
where we know that

r = radial distance of the point

also for the linear speed of the point on the disc is given as

`v = r \omega`

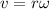
so we know that

distance of point Q is double the distance of point P

so we will have

`a_Q = 2 a_P`

`v_Q = 2 v_P`