The sum of
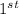 five terms of the series is 121.
five terms of the series is 121.
Option - B
SOLUTION:
Given that, we have to find the sum of the first five terms of the geometric series 1 + 3 + 9 + ...
We already know, first three terms, let us find next two terms also.
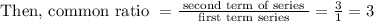
Now, we know that,
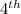 term is
term is
 term multiplied by the common ratio so,
term multiplied by the common ratio so,

And,
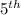 term is
term is
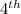 term multiplied by common ratio. So,
term multiplied by common ratio. So,
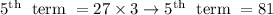
Now, sum of first five terms =
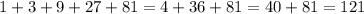
Hence, the sum of
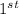 five terms of the series is 121.
five terms of the series is 121.