Question

What is instantaneous acceleration?

A. acceleration in a straight line
B. acceleration that happens very quickly
C. acceleration over a long period of time
D. acceleration at a specific moment in time

Answer 1

D. acceleration at a specific moment in time

Step-by-step explanation:

The acceleration of a body is defined as the change in velocity of the body in the given time.

If a body has initial velocity 'u' and final velocity 'v' over a time interval 't', then the acceleration of the body is given by the relation,

a = v -u / t

Consider a body travel from time ' t = o' to ' t = n'. In such cases, the acceleration of the body is given by average acceleration, which is the change in velocity of the body in that particular interval of time.

Aₐ = ΔV / Δt

But at each instant of time from t = 0 to n, the object experience different velocity. Therefore, the acceleration of the body at specific time, t₁, t₂, t₃, etc. is given by,

`a_(i)= \lim_(n \to0) \frac{\delta{v}}{\delta{t}_(i)}`

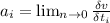
This is the instantaneous acceleration at the particular instant of time.