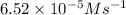Answer :
(a) The rate law for the reaction is:
![\text{Rate}=k[OCl^-]^1[I^-]^1](https://img.qammunity.org/2020/formulas/chemistry/high-school/wqxh05a25667xbtfq574qfaao2qxf1i0k0.png)
(b) The value of rate constant is,
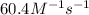
(c) rate of the reaction is
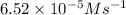
Explanation :
Rate law : It is defined as the expression which expresses the rate of the reaction in terms of molar concentration of the reactants with each term raised to the power their stoichiometric coefficient of that reactant in the balanced chemical equation.
For the given chemical equation:
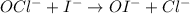
Rate law expression for the reaction:
![\text{Rate}=k[OCl^-]^a[I^-]^b](https://img.qammunity.org/2020/formulas/chemistry/high-school/wqo7oy9fdcxyq3djdt5zgx4e0c91i1yrnv.png)
where,
a = order with respect to
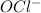
b = order with respect to
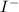
Expression for rate law for first observation:
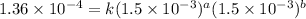 ....(1)
....(1)
Expression for rate law for second observation:
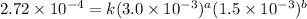 ....(2)
....(2)
Expression for rate law for third observation:
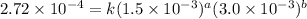 ....(3)
....(3)
Dividing 1 from 2, we get:

Dividing 1 from 3, we get:
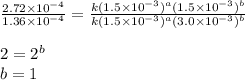
Thus, the rate law becomes:
![\text{Rate}=k[OCl^-]^a[I^-]^b](https://img.qammunity.org/2020/formulas/chemistry/high-school/wqo7oy9fdcxyq3djdt5zgx4e0c91i1yrnv.png)
a = 1 and b = 1
![\text{Rate}=k[OCl^-]^1[I^-]^1](https://img.qammunity.org/2020/formulas/chemistry/high-school/wqxh05a25667xbtfq574qfaao2qxf1i0k0.png)
Now, calculating the value of 'k' (rate constant) by using any expression.
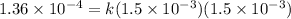
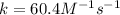
Now we have to calculate the rate for a reaction when concentration of
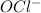 and
and
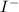 is
is
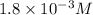 and
and
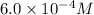 respectively.
respectively.
![\text{Rate}=k[OCl^-][I^-]](https://img.qammunity.org/2020/formulas/chemistry/high-school/ayn0r2bnpsx2l2w7tpacni4e32s84egwyy.png)

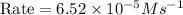
Therefore, the rate of the reaction is