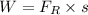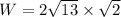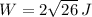Answer:
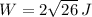
Step-by-step explanation:
Given that:
Relation of force with position,
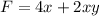 N
N
Initial position of the object,
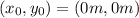
second position of the object,
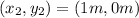
final position of the object,

Now, from the schematic we get the displacement as:
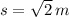
Now we calculate :
The force in X direction for initial displacement


The force in Y direction for initial displacement
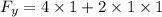
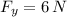
Now the resultant force in the direction of displacement:
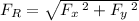
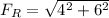
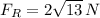
Therefore work: