Answer:
-2.915,0.00362
Explanation:
Given that a study was recently conducted at a major university to determine whether there is a difference in the proportion of business school graduates who go on to graduate school within five years after graduation and the proportion of non-business school graduates who attend graduate school.
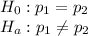
(Two tailed test for comparison of two proportion at 8% significance level)
we have
business school non business school proportion
70 400 0.175
100 500 0.20
p difference =-0.025
Std error of p difference
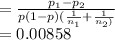
a) Z statistic = p diff/Std error =-2.915
b) p value = 0.00362
Since p <0.08 we reject null hypothesis.
There is significant difference between the two proportions.