Answer:
Approximate length of the diagonal fencing needed is 45.25 cm.
Explanation:
Here, each side of the square shaped land = 32 ft
Now, the diagonal fencing is made in side the plot so that the land is divided into two right triangles.
In the triangle:
Base side of the triangle = 32 cm
Perpendicular length of the triangle = 32 cm
Let us assume the hypotenuse of the triangle = k cm
⇒The length of the diagonal in the square = k cm
by PYTHAGORAS THEOREM in a right angled triangle:
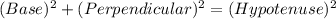
⇒
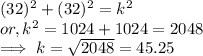
or, k = 45.25 cm
Hence,the approximate length of the diagonal fencing needed to fence the square land is 45.25 cm.