Answer:
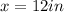
Explanation:
the formula for the volume of a square pyramid is:
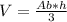
where
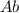 is the area of the base (the area of the square)
is the area of the base (the area of the square)
and
 if the height of the pyramid
if the height of the pyramid
---------
the length of the side of the square is
 , so the area of the base of the pyramid is:
, so the area of the base of the pyramid is:

and we already know the height in terms of x:
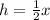
and the Volume:
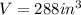
so we subtitute all of this in the formula for volume:
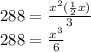
and finally we clear for

![288*6=x^3\\1728=x^3\\\sqrt[3]{1728}=x\\12=x](https://img.qammunity.org/2020/formulas/mathematics/middle-school/hje91sil4tgopfpbeadxeu7uhtowp9s9h6.png)
the value of x is 12 inches.