Answer:
-6327.45 Joules
650.375 Joules
378.47166 N
Step-by-step explanation:
h = Height the bear slides from = 15 m
m = Mass of bear = 43 kg
g = Acceleration due to gravity = 9.81 m/s²
v = Velocity of bear = 5.5 m/s
f = Frictional force
Potential energy is given by

Change that occurs in the gravitational potential energy of the bear-Earth system during the slide is -6327.45 Joules
Kinetic energy is given by

Kinetic energy of the bear just before hitting the ground is 650.375 Joules
Change in total energy is given by
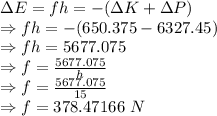
The frictional force that acts on the sliding bear is 378.47166 N