Question

A 40.0 mA current is carried by a uniformly wound air-core solenoid with 435 turns, a 16.5 mm diameter, and 12.5 cm length. (a) Compute the magnetic field inside the solenoid. µT (b) Compute the magnetic flux through each turn. T·m2 (c) Compute the inductance of the solenoid. mH (d) Which of these quantities depends on the current? (Select all that apply.) magnetic field inside the solenoid magnetic flux through each turn inductance of the solenoid

Answer 1

55.68 μT

`1.19058* 10^(-8)\ Tm^2`

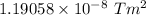
0.12 mH

Magnetic flux and magnetic field

Step-by-step explanation:

i = Current = 40 mA

N = Number of turns = 435

D = Diameter of solenoid = 16.5 mm

l = Length of wire = 12.5 cm

A = Area of solenoid =
`\pi \left((D)/(2)\right)^2`

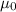`\mu_0` = Vacuum permeability =
`4\pi * 10^(-7)\ H/m`

Magnetic field of a solenoid is given by

`B=(\mu_0Ni)/(l)\\\Rightarrow B=(4* 10^(-7)* 435* 4* 10^(-3))/(12.5* 10^(-2))\\\Rightarrow B=0.00005568\ T=55.68* 10^(-6)\ T=55.68\ \mu T`

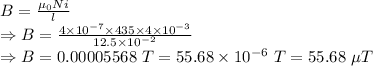
The magnetic field inside the solenoid is 55.68 μT

Magnetic flux is given by

`\phi=BA\\\Rightarrow \phi=0.00005568* \pi* \left((16.5* 10^(-3))/(2)\right)^2\\\Rightarrow \phi=1.19058* 10^(-8)\ Tm^2`

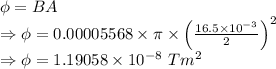
The magnetic flux through each turn is
`1.19058* 10^(-8)\ Tm^2`

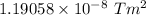
Inductance of solenoid is given by

`L=(N\phi)/(i)\\\Rightarrow L=(435* 1.19058* 10^(-8))/(40* 10^(-3))\\\Rightarrow L=0.00012\ H=0.12\ mH`

The inductance of the solenoid is 0.12 mH

As can be seen from the formulae above magnetic flux and magnetic field depend on current.