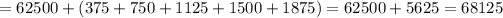Question

Sam deposits 12,500 each year into a retirement account with a %3 simple interest rate. If he deposits the same amount each year, how much money will he have at the end of his fourth year?

Answer 1

the Answer is $52,250.

Explanation:

Use A=P + I to determine the total amount earned on each years investment.

Answer 2

The money in bank after 4 years will be
`\bold{\$68125}`

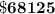
Solution:

Given, Sam deposits 12,500 each year into a retirement account with a
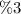`\%3` simple interest rate.

He deposits the same amount each year, we have to find the amount of money will he have at the end of his fourth year.

We know that,
`\text { Simple Interest }=\frac{\text {Amount } * \text { Rate } * \text { Time}}{100}`

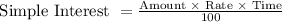
So, now let us find S.I after
`1^{\mathrm{st}} \text{ year } =(12500 * 3 * 1)/(100)=125 * 3=375`

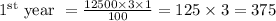
Then, after
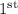`1^{\text {st }}` year he adds 12,500 again, which means amount doubles
`\rightarrow` S.I also doubles as rate and time of 1 year gap are constant.

Then, S.I for
`2^{\text {nd}} \text {year }=375 * 2=750`

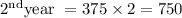
Amount and corresponding S.I for 4 years will be,

`\begin{array}{l}{12500 \rightarrow 375} \\\\ {25000 \rightarrow 750} \\\\ {37500 \rightarrow 1125} \\\\ {50000 \rightarrow 1500} \\\\ {62500 \rightarrow 1875}\end{array}`

Now, total balance = amount after
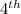`4^(th)` year + sum all simple interests made up to now.

Total balance
`= 62500 + (375 + 750 + 1125 + 1500 + 1875) = 62500 + 5625 = 68125`