Answer: (17.42, 20.78)
Explanation:
As per given , we have
Sample size : n= 9
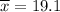 years
years
Population standard deviation is not given , so it follows t-distribution.
Sample standard deviation : s= 1.5 years
Confidence level : 99% or 0.99
Significance level :
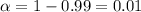
Degree of freedom : df= 8 (∵df =n-1)
Critical value :
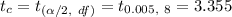
The 99% confidence interval for the population mean would be :-
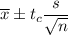
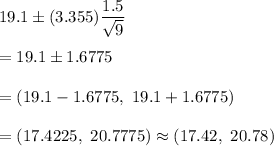
Hence, the 99% confidence interval for the population mean is (17.42, 20.78) .