Answer:

Explanation:
We want to find the polynomial function whose zero are -1 and 4i.
Recall that non-real zeros come in pairs.
There if 4i is a zero, then the conjugate -4i is also a zero.
Therefore the factors of this polynomial are:
x+1, x+4i, and x-4i are factors of the given polynomial.
Let f(x) be the required polynomial, then we can write the polynomial in factored form as:
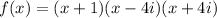
We expand the conjugate pair using difference of two squares to get:
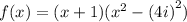
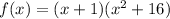
Expand further using the distributive property to get:
