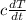Question

How do you do this question?

Answer 1

The required relation is,

`\frac {dV}{dt}` =
`c{(dT)/(dt)}`

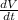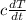
Explanation:

We know that for a certain amount of dry gas when the pressure is kept constant it's volume V and temperature T is related by the function,

V = cT -----------------------(1) [where "c" is a constant]

So, in that case rate of change of volume(V) with respect to time (t),

=
`\frac {dV}{dt}`

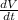
will be equal to ,

`c{(dT)/(dt)}`

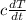
where
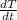`\frac {dT}{dt}` is equal to rate of change of temperature (T) with respect to time (t) and c is the constant stated before.

So, the required relation is,

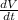`\frac {dV}{dt}` =
`c{(dT)/(dt)}`