Question

Pls can someone help me and have it right

Answer 1

Problem 1:

a. x=2

b. x=3

c. x=1

Problem 2:

A multiplication equation to hold the table true:

`18*3=54`

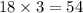
A division equation to hold the table true:

`(54)/(3) =18`

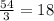
Explanation:

Given in problem 1:

(a). The equation is
`(x)/(6) > 1`

It holds true for all values of
`x> 6`.

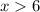Let us say
`x=12`,

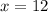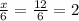`(x)/(6)=(12)/(6)  =2` which is greater than 1.

(b). The equation is
`(x)/(6) < 1`

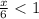
It holds true for all values of
`x< 6`.

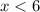Let us say
`x=3`

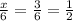`(x)/(6) =(3)/(6) =(1)/(2)` which is less than 1.

(c). The equation is
`(x)/(6) = 1`

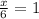
It holds true for only
`x= 6`.

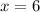Let us say
`x=6`,

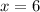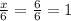`(x)/(6) =(6)/(6)=1` which is equal to 1.

Problem 2:

A multiplication equation to hold the table true:

`18*3=54`

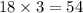
A division equation to hold the table true:

`(54)/(3) =18`

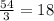
Therefore these are the values which hold true to the equation in problem 1 and 2.