Question

Coach henry tied a rope to the top of a 6 ft pole and

secured the rope to the ground 6 ft from the base of
the pole. How long is the rope?

​

Answer 1

8.49 feet

Explanation:

From this scenario, a right triangle is created, where,

Pole (6ft) is left side of triangle,

Ground Distance (6ft) from pole to where rope is secured is the base of the triangle

Hence, the rope itself is the HYPOTENUSE of the triangle (hypotenuse is the side oppose of 90 degree angle -- this angle is created with ground and pole)

Now that we know both legs of the triangle are 6 each and we need to find hypotenuse, we use the Pythagorean Theorem to solve this:

Pythagorean Theorem is Hypotenuse ^2 = Leg^2 + AnotherLeg^2

Here,

Leg = 6

AnotherLeg = 6

So we write and equation and solve for Hypotenuse (h):

`h^2=6^2 + 6^2\\h^2 = 36 + 36\\h^2 = 72\\h=√(72) \\h=8.49`

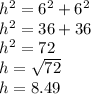
The rope's length is 8.49 feet (rounded to 2 decimal places)