Answer:
The nth term of the given sequence is
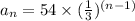 .
.
Explanation:
Here, the first term of the sequence = 54
Second term = 18
Third term = 6
Now,
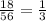
and,
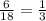
as
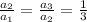
Hence, the terms of the given sequence are in GEOMETRIC PROGRESSION with common ratio = 1/3
Now, the nth term in GP is given as
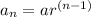
So here,
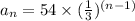
Hence, nth term of the given sequence is
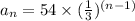 .
.