Question

Choose two of the following choices that can represent the nth term of the sequence: 4, 7, 10, 13, ...

a_n=4n+3, n≥1

a_n=3n+4, n≥1

a_n=4+(n-1)*3, n≥1

a_n=3n+1, n≥1

a_n=3+(n-1)*4, n≥1

Answer 1

Answer:
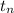`t_(n)` = 3n + 1 , n≥ 1

Explanation:

The common difference of the sequence = 3 , so it is an arithmetic sequence.

The formula for the nth term of an arithmetic sequence is given as:

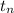`t_(n)` = a + (n-1)d

substituting the values of a and d , we have

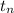`t_(n)` = 4 + (n-1) X 3

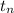`t_(n)` = 4 + 3n - 3

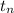`t_(n)` = 1 + 3n

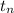`t_(n)` = 3n + 1 , n≥ 1