Question

A0.382 kg glass of milk is on

a counter with us = 0.125. How
much force does it take to set it
in motion? Watch your sig figs.
(Unit = N)

Answer 1

.468 is the correct answer on Acellus

Answer 2

The force here must be greater than the frictional force and that is
`= f=\mu * N =0.4655\ N`

Step-by-step explanation:

Given values,

Mass of the milk (m)
`= 0.382\ kg`

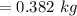
Co-efficient of static friction
`=\mu= 0.125`

To calculate force required, for the glass of milk to set it in motion.

We have to calculate the frictional forces (f) acting over it.

And the force must be greater than or equivalent to that force.

We know that
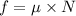`f=\mu * N`,and
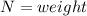`N= weight` N=Normal force,here it is equivalent to the weight acting on the body.

Weight (w)
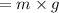`=m * g`, and g
`=9.8ms^(2)`

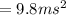
So
`f=\mu * N = 0.125* 0.382 * 9.8 =0.4655\ N`

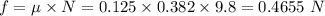
The force required to set the glass of milk in motion
`=0.4655\ N`