Question

What is the area of the unshaded region of the rectangle? part 1. Show work please​

Answer 1

`\displaystyle 96\:m^2`

Explanation:

You can easily solve this using Pythagorean Triplets:

`\displaystyle 3, 4, 5 \\ 6, 8, 10 \\ 9, 12, 15 \\ \boxed{12, 16, 20}`

The shorter leg is twelve metres long. Now you must find the area of this right triangle:

`\displaystyle (hb)/(2) = A\:OR\:(1)/(2)hb = A \\ \\ ([16][12])/(2) = (192)/(2) = 96\:OR\:(1)/(2)[16][12] = (1)/(2)[192] = 96`

You see how swift that was?

I am joyous to assist you at any time.

Answer 2

96 m²

Explanation:

The unshaded region is a right triangle.

The area (A) of the triangle is calculated as

A =
`(1)/(2)` bh ( b is the base and h the perpendicular height )

Calculate b using Pythagoras' identity in the right triangle

b² + 16² = 20²

b² + 256 = 400 ( subtract 256 from both sides )

b² = 144 ( take the square root of both sides )

b =
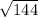`√(144)` = 12

Thus area of unshaded region is

A =
`(1)/(2)` × 12 × 16 = 6 × 16 = 96 m²