Question

The product of two positive consecutive integers is 240. Find the integers.

Answer 1

Answer: 15 & 16

Explanation:

240/15=16

15x16=240

Answer 2

The product of two positive consecutive integers is 240. Then the integers are 15 and 16

Solution:

Given that product of two positive consecutive integers is 240

Let the first number be "x"

Since the numbers are consecutive, then the second number be "x + 1"

According to given information,

Product of "x" and "x + 1" = 240

`x * (x + 1) = 240\\\\x^2 + 1x = 240\\\\x^2 + x -240 = 0`

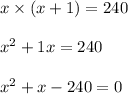
On factorizing we get,

Find two numbers which add up to 1 and when multiplied gives -240

16 and -15 are the two numbers which satisfies the above condition

Hence the factors are:

(x - 15)(x + 16) = 0

Equating to 0 we get,

(x - 15) = 0

x = 15

And (x + 16) = 0

x = -16 ; Since its given that positive integers. Negative value is ignored

Hence "x" = 15

Hence the first integer is "x" = 15

And the second integer is "x + 1" = 15 + 1 = 16