Question

RajDee Furniture Company (RFC) buys and sells office furniture. The company buys chairs from a manufacturer for $40 per unit. Order costs are $200 per order and there is a lead time of 10 days for each order to arrive from the manufacturer to RFC warehouse. Inventory carrying cost for RFC is 10%. Average yearly demand for the chairs is 40,000 units. Answer the following questions, assuming there is no uncertainty at all about the demand or the lead time.1) How many units should RFC order each time?2) What would average inventory be if RFC orders this quantity every time?3) If average lead time went up from 10 to 15 days, what will happen to EOQ?

Answer 1

Answer:The answer( 1)Re-order level 600,000 unit,(2) Average stock 21,000 unit, (3) it will reduce the EOQ because, it will delay the time when the goods should be available for use.

Step-by-step explanation:

Demand = 40,000 unit per annum

Ordering cost = $200 per order

Carrying cost = 10% × $40 = 10/100 × 40 = $4

EOQ = √2DCO /CC

Where CO = ordering cost per order

D = Demand per annum

CC = carrying cost per item per annum

EOQ = √2 × 40,000 × 200/4

= √16,000,000/4

= √4,000,000

= 2,000 unit

(1) Re- order level = Maximum usage × Maximum lead time

= 40,000 × 15

= 600,000 unit

(2) Averagestock = Maximum stock level + Minimum stock level / 2

= 40,000 + 2000/2

= 42,000/2

= 21,000

(3) The EOQ will be reduced because, it will delay the time when the goods should be available for use.

Answer 2

(1) 2,28 units

(ii) 1,414 units

(iii) Minimum stock is less than EOQ.

Step-by-step explanation:

(1) Units Ordered each time

`Economic\ order\ Quantity=\sqrt{(2* A* O)/(C) }`

where,

A = Annual Requirement =40,000 Units

O = Ordering Cost = $200 Per unit

Minimum Stock for lead time:

= (40,000 Units × 10) ÷ 365

= 1096 (Approximately)

C=Annual Carrying cost per unit = $40 × 10% × 1/2

= 2

`Economic\ order\ Quantity=\sqrt{(2* 40,000* 200)/(2) }`

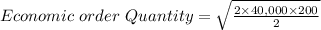
= 2828 Units

(2) Average Inventory = EOQ ÷ 2

= 2828 Units ÷ 2

= 1,414 Units

(3) If the Lead time Increase 10 to 15 days:

Minimum Stock Need to be Maintained:

= Avg Daily Demand × Lead time

= (40,000 Units ÷ 365) × 15

= 1,644 Units

Minimum Stock is Less the EOQ , then Increasing Lead time to 15 Days Does not Have effect on EOQ.