Slope intercept form of a line passing through (-1, -2) and (1, -4) is
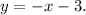
Solution:
We have to find the equation of a line in slope intercept form.
Given that
Line is passing through point (− 1, − 2) and (1, − 4).
Equation of line passing through point
 and
and
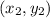 is given by,
is given by,
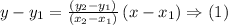
In our case

Substituting given value in (1) we get ,
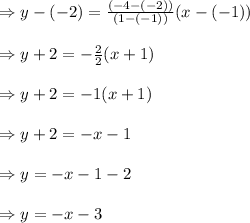
Hence slope intercept form of a line passing through (-1, -2) and (1, -4) is
