Question

The overall reaction and equilibrium constant value for a hydrogen-oxygen fuel cell at 298 K is given below. 2 H2(g) + O2(g) → 2 H2O(l) K = 1.34 ✕ 1083 (a) Calculate ℰ° and ΔG° at 298 K for the fuel-cell reaction. ℰ° V ΔG° kJ (b) Predict the signs of ΔH° and ΔS° for the fuel-cell reaction. ΔH°: positive negative ΔS°: positive negative (c) As temperature increases, does the maximum amount of work obtained from the fuel-cell reaction increase, decrease, or remain the same? Explain. Since ΔS is , as T increases, ΔG becomes more . Therefore, the maximum work obtained will as T increases.

Answer 1

(a) ΔG° = -474 kJ/mol; E° = 1.23 V

(b) ΔH° negative; ΔS° negative

(c) Since ΔS is negative, as T increases, ΔG becomes more positive. Therefore, the maximum work obtained will decrease as T increases.

Step-by-step explanation:

Let's consider the following reaction.

2 H₂(g) + O₂(g) → 2 H₂O(l)

with an equilibrium constant K = 1.34 × 10⁸³

(a) Calculate E° and ΔG° at 298 K for the fuel-cell reaction.

We can calculate the standard Gibbs free energy (ΔG°) using the following expression:

ΔG° = - R × T × lnK

ΔG° = - 8.314 × 10⁻³ kJ . mol⁻¹.K⁻¹ × 298 K × ln 1.34 × 10⁸³ = -474 kJ/mol

To calculate the standard cell potential (E°) we need to write oxidation and reduction half-reactions.

Oxidation: 2 H₂ ⇒ 4 H⁺ + 4 e⁻

Reduction: O₂ + 4 e⁻ ⇒ 2 O²⁻

The moles of electrons (n) involved are 4.

We can calculate E° using the following expression:

`E\°=(0.0591V)/(n) .logK\\E\°=(0.0591V)/(4) .log1.34 * 10^(83)=1.23V`

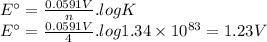
(b) Predict the signs of ΔH° and ΔS° for the fuel-cell reaction. ΔH°: positive negative ΔS°: positive negative

The standard Gibbs free energy is related to the standard enthalpy (ΔH°) and standard entropy (ΔS°) through the following expression:

ΔG° = ΔH° - T.ΔS°

Usually, the major contribution to ΔG° is ΔH°. So, if ΔG° is negative (exergonic), ΔH° is expected to be negative (exothermic).

The entropy is related to the number of moles of gases. There are 3 gaseous moles in the reactants and 0 in the products, so the final state is predicted to be more ordered than the initial state, resulting in a negative ΔS°.

(c) As temperature increases, does the maximum amount of work obtained from the fuel-cell reaction increase, decrease, or remain the same?

The maximum amount of work obtained depends on the standard Gibbs free energy.

wmax = ΔG° = ΔH° - T.ΔS°

Since ΔS is negative, as T increases, ΔG becomes more positive. Therefore, the maximum work obtained will decrease as T increases.