Answer:
Length and width = 2.71 cm and height = 5.43 cm
Explanation:
Let the length of the jewelry box = x
Let the width = x [box is square, so length and width are same]
Let the height = y
Given volume = 40 cm³
Therefore, V = x²y
x²y = 40
y =
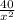
Cost of Nickle plating on top and bottom = $2 per cm²
Cost of copper plating on sides = $1 per cm²
Cost = 2(Areas of top and bottom) + 1(Areas of sides)
= 2(x² + x²) + 1(xy + xy + xy + xy)
= 4x² + 4xy
= 4x² = 4x
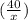
f(x) = 4x² +

f'(x) = 8x -
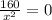
f"(x) =
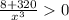
f'(x) = 0
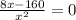
x³ = 20
x = ∛20
x = 2.71 cm
x²y = 40
∛400y = 40
y =
![\frac{40}{\sqrt[3]{400} }](https://img.qammunity.org/2020/formulas/mathematics/college/kjtm6k7r8kyc82x7kmpzw11tl8p1o1je3s.png)
=
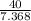
y = 5.4288 ≈ 5.43 cm
Length and width = 2.71 cm and height = 5.43 cm