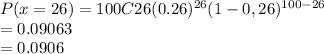Question

Suppose that the prevalence of a certain type of tree allergy is 0.26 in the general population. If 100 people randomly chosen from this population are tested for this allergy, what is the probability that exactly 26 of them will have this allergy? Please write your answer as a decimal, precise to at least four decimal places.

Answer 1

The probability that exactly 26 out of 100 randomly selected will have this allergy is P=0.0906.

Explanation:

We can model this as a binomial distribution with parameters:

Size n=100

Probability p=0.26.

We can calculate the probability of getting a sample of exactly 26 positives as:

`P(X=k)=(n!)/(k!(n-k)!) p^k(1-p)^(n-k)\\\\P(X=26)=(100!)/(26!(100-26)!) 0.26^(26)(1-0.26)^(100-26)\\\\P(X=26)=(6.9957*10^(23))*(6.1561*10^(-16))*(2.1045*10^(-10))\\\\P(X=26)=(6.9957*6.1561*2.1045)*10^(23-16-10)\\\\P(X=26)=90.6329*10^(-3)=0.0906`

Answer 2

0.0906

Explanation:

Given that the prevalence of a certain type of tree allergy is 0.26 in the general population

X- the no of persons having tree allergy is binomial since each persons is independent of the other and there are two outcomes.

Here n = 100

Required probability = the probability that exactly 26 of them will have this allergy

=
`P(x=26)=100C26(0.26)^(26) (1-0,26)^(100-26)\\ =0.09063\\=0.0906`