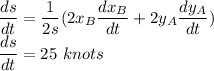Question

Two boats leave the same port at the same time, with boat A traveling north at 15 knots (nautical miles per hour) and boat B traveling east at 20 knots. How fast is the distance between them changing when boat A is 30 nautical miles from port?

Answer 1

The chance in distance is 25 knots

Step-by-step explanation:

The distance between the two particles is given by:

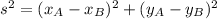`s^2 = (x_A - x_B)^2+(y_A - y_B)^2` (1)

Since A is traveling north and B is traveling east we can say that their displacement vector are perpendicular and therefore (1) transformed as:

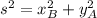`s^2 = x_B^2+y_A^2` (2)

Taking the differential with respect to time:

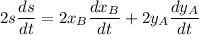`\displaystyle{2s(ds)/(dt)= 2x_B(dx_B)/(dt)+2y_A(dy_A)/(dt)}` (3)

where
`\displaystyle{(dx_B)/(dt)}=v_B` and
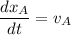`\displaystyle{(dx_A)/(dt)}=v_A` are the respective given velocities of the boats. To find
`s` and
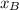`x_B` we make use of the given position for A,
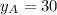`y_A=30`, the Pythagoras theorem and the relation between distance and velocity for a movement with constant velocity.

`\displaystyle{y_A = v_A\cdot t\rightarrow t = (y_A)/(v_A)=(30)/(15)=2 h`

with this time, we know can now calculate the distance at which B is:

`\displaystyle{x_B = v_B\cdot t= 20 \cdot 2 = 40\ nmi`

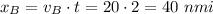
and applying Pythagoras:

`\displaystyle{s = √(x_B^2+y_A^2)=√(30^2 + 40^2)=√(2500)=50}`

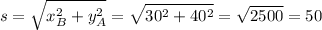
Now substituting all the values in (3) and solving for
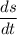`\displaystyle{(ds)/(dt) }` we get:

`\displaystyle{(ds)/(dt) = (1)/(2s)(2x_B(dx_B)/(dt)+2y_A(dy_A)/(dt))}\\\displaystyle{(ds)/(dt) = 25 \ knots}`