Answer:
Question 1: 20
Question 2: 27
Explanation:
Question 1:
Given a sequence is defined by the explicit definition
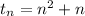
Therefore, the 4th term i.e.
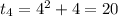 (Answer)
(Answer)
So, the third option is correct.
Question 2:
Given a sequence is defined by the explicit definition
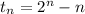
Therefore, the 5th term i.e.
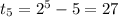 (Answer)
(Answer)
So, the third option is correct.