The least common denominator of two fraction is the least common multiple of the two denominator.
The least common multiple of 4 and 7 is their product 28, because 4 and 7 have no common factor.
Now, we need to manipulate both fractions so that they have denominator 28. Recalling that you can multiply numerator and denominator of a fraction by the same number without affecting its value, we have
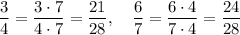
So, the sum becomes
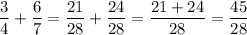
In fact, when two fractions have the same denominator, you can directly sum their numerators.